Poker Dice Probability Full House

Poker dice is played by simultaneously rolling five dice show that a P no two from ECE 101 at Iowa State University. Study Resources. Poker dice is played by simultaneously rolling five dice. Show that (a) P. 0193 (g) P five alike = 0. 0008 Note that a full house is three of one number plus a pair of another number. I'm making a dice game. There are 5 dice in which I have all the values for and I need to determine if they make up a full house (3 of one and 2 of another), small straight (1-4, 2-6 or 3-6) or a large straight (1-5, 2-6).

• Poker Probability Formulas
• Multiple Dice Probability
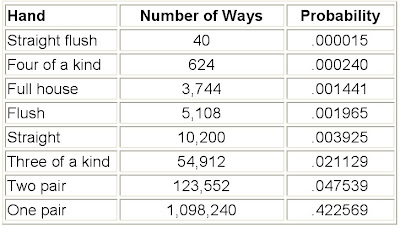
• Poker Dice Probability Full House 2
• Craps Dice Probability
• Poker Dice Online
• Probability In Poker

The odds of flopping a full house with a pocket pair is 0.98% or 1 in 102

Definition of Full House (also known as a Boat or Full Boat) –

Three cards of one rank and two cards of another. (I.e. three of a kind and a pair made at the same time).

Example – AdAhAsKcKh

Aces Full of Kings the strongest Full House hand in poker and is also referred to as “Aces Full”.

Odds of Making a Full House on the Flop

Full Houses are rare in poker. Hitting on the flop is hence not a common occurrence.

Odds of flopping a Full House with any starting hand = 0.14%

Poker Probability Formulas

Odds of flopping a Full House with any unpaired hand = 0.09%

Odds of flopping a Full House with a pocket pair = 0.98%

To put that into context;

A pocket pair will hit a Full House roughly once every 100 flops. An unpaired hand will hit a Full House once every 1,000 flops. Ignoring the exact starting hand, we should expect to flop (on average) one Full House for every 714 flops we see.

Assuming about 25% of our holdings see a flop, we should expect to flop a Full House once every 2,857 hands.

Of course, the above calculation is just an average, sometimes we’ll flop more often, other times less frequently.

The odds of making a Full House or better should not change drastically, because there simply aren’t that many better hands than a Full House.

Odds of flopping a Full House or better with any starting hand = 0.17

Odds of flopping a Full House or better with AKo = 0.1%

Odds of flopping a Full House or better with T9s = 0.12%

Odds of flopping a Full House or better with 88 = 1.22%

We can see that a pocket pair is by far our best shot at being able to make a Full House or better, hitting roughly 10 times as often as unpaired hands.

Odds of Making a Full House on the Later Streets

There are generally two main types of Full House draw postflop -

1) We have a Two Pair and are drawing for one of our Pairs to make trips.
2) We have Three of a Kind and are hoping that we make a Pair. https://golmaine.netlify.app/slot-machine-handle-sound-effect.html.

Scenario 1 is the easiest since we know exactly which cards we are waiting to Pair.

Example 1

Flop: KT7
Our Hand: KT

We already have two pair and can either catch a King or a Ten to make a Full House. There are two Tens and two Kings left in the deck, giving us a total of four possible outs.

Odds of hitting on the turn = 4/47 = 0.0851 or roughly 8.5%

Odds of hitting on the river = 4/46 = 0.087 or roughly 8.7%

Odds of hitting on the turn or river (we’ll calculate Odds of not hitting then deduct from 100).

Odds of not hitting on the turn = 43/47

Odds of not hitting on the river = 42/46

Odds of not hitting on the turn or river = 43/47 * 42/46 = 0.8353 or roughly 83.5%

Odds of hitting is, therefore (100 – 83.5) roughly 16.5%

Scenario 2 is a little bit tougher since we can also make a Full House if the board pairs.

Example 2

Flop KT7
Our Hand: TT

We already have Three of a Kind here and simply need any other card to pair. Either the King or Seven could pair for a total of 6 outs. It’s also worth considering that we can catch the case Ten (one out) to make Quads.

Assuming the turn bricks, it still puts another card out there that can pair by the river. In other words, unlike the previous example, we are guaranteed to pick up additional outs on the turn if we don’t hit.

Odds of hitting on the turn (including Quads) = 7/47 = 0.1489 or roughly 15%

Odds of hitting on the river (including Quads) = 10/46 = 0.2174 or roughly 21.7%

Multiple Dice Probability

We are noticeably more likely to hit our Full House going from turn to river.

Odds of hitting on the turn or river (we’ll calculate Odds of not hitting then deduct from 100).

Poker Dice Probability Full House 2

Odds of not hitting on the turn (including Quads) = 40/47

Odds of not hitting on the river (including Quads) = 36/46

Odds of not hitting on the turn or river = 40/47 * 36/46 = 0.6660 or roughly 66.6%

Odds of hitting by the river are hence (100-66.6) roughly 33.4%

Implied Odds Analysis of a Full House

Like most other strong hands, the value of the implied odds depends much on the relative strength of the holding. There are strong Full Houses and weak Full Houses.

Keep in mind the following -

Overfull/Underfull – The terms “overfull” and “underfull” are used to describe nut and non-nut Full Houses, respectively. For example, on an 8-8-7 board, 87 is the overfull while 77 is the underfull.

In most cases, it’s just a cooler if these hands run into each other, but technically the two-card overfull carries better-implied odds than the two-card underfull.

One-Card/Two-Card – The issue of overfull/underfull becomes even more pronounced when we are dealing with one-card Full Houses (i.e. using only one of our hole cards to create the hand).

Craps Dice Probability

On a TT77x any Tx is the overfull while any 7x is the underfull. Seeing as it’s simply much more common that any player would have a Full House on a double-paired texture, the one-card underfull needs to be treated with caution.

The one-card underfull is actually more likely to generate reverse implied odds than implied odds due to the high possibility it is dominated if Villain wants to get the stacks in.

Poker Dice Online

Basic Strategy Advice

Full Houses are ultra-strong hands in Hold’em and should nearly always be stacked off for 100bb stacks. The only exception is really the one-card underfull. This is still a relatively strong hand, but we’ll likely try and avoid getting all of the stacks in when facing aggression.

Probability In Poker